How many pattern block rhombuses would 10 triangles create sets the stage for a deeper understanding of geometric relationships and the practical applications of pattern blocks. This seemingly simple question delves into the fundamental principles of area equivalence and shape conversion, revealing a hidden world of mathematical patterns within the seemingly playful realm of pattern blocks.
Pattern blocks, with their distinct shapes and vibrant colors, are often used in early childhood education to introduce fundamental geometric concepts. However, beneath the surface of these seemingly simple shapes lies a rich tapestry of mathematical relationships. This exploration will delve into the relationship between triangles and rhombuses, demonstrating how a specific number of triangles can be transformed into rhombuses, and how this conversion can be applied to various practical scenarios.
Understanding the Shapes

The journey to discover how many rhombuses can be made from 10 triangles begins with understanding the fundamental shapes involved – the triangle and the rhombus. These are not just random geometric shapes; they are building blocks in the world of pattern blocks, each with its own unique properties.
The Relationship Between a Triangle and a Rhombus
The relationship between a triangle and a rhombus in pattern blocks is one of transformation. A rhombus can be formed by combining two equilateral triangles.
- Equilateral Triangle: An equilateral triangle is a triangle with all three sides equal in length and all three angles measuring 60 degrees. The pattern block triangle is an equilateral triangle.
- Rhombus: A rhombus is a quadrilateral with all four sides equal in length. The pattern block rhombus is a special kind of rhombus called a “diamond.” It has two acute angles (less than 90 degrees) and two obtuse angles (greater than 90 degrees).
The Concept of Area
The area of a shape is the amount of space it occupies. In the context of pattern blocks, we can think of the area as the number of smaller units that fit inside the shape.
The area of a shape is the amount of two-dimensional space it occupies.
The area of a pattern block triangle is considered to be one unit. This means that we can measure the area of any shape formed by pattern blocks by counting the number of triangles it contains.
Visual Representation
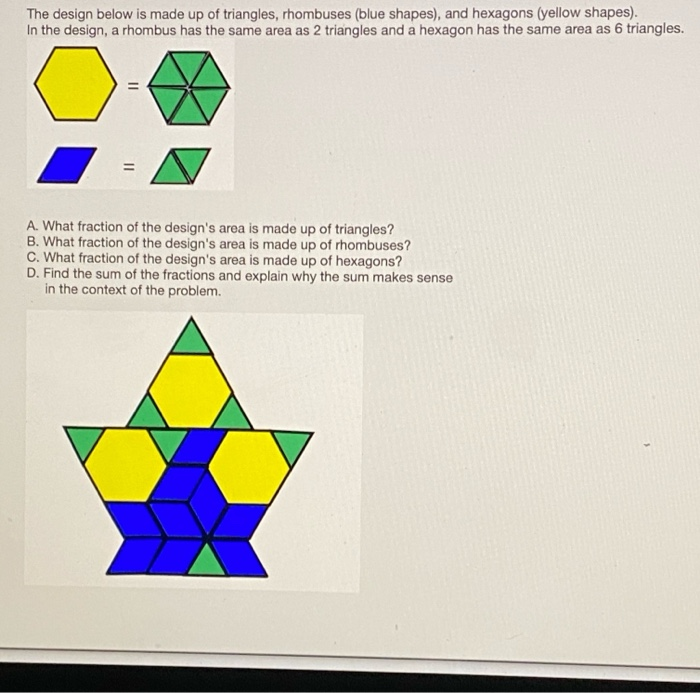
Visualizing the relationship between triangles and rhombuses formed from them is crucial for understanding the pattern and relationship between these shapes.
Let’s begin by understanding how a rhombus is formed from triangles. A rhombus is a quadrilateral with four equal sides. It can be formed by joining two equilateral triangles along their bases, creating a rhombus with a larger area. The area of a rhombus is determined by its base and height, while the area of a triangle is determined by its base and height.
Visual Representation of Rhombus Formation
Imagine an equilateral triangle. Now, imagine another equilateral triangle of the same size placed next to it, sharing the same base. When you join these two triangles, you form a rhombus. The base of each triangle becomes one of the sides of the rhombus, and the height of each triangle becomes the height of the rhombus. This arrangement demonstrates the direct relationship between the number of triangles and the resulting rhombus.
Relationship between Triangles and Rhombuses
The number of triangles directly influences the number of rhombuses that can be formed. Each pair of triangles creates one rhombus. This relationship is reflected in the table below, which showcases the pattern as the number of triangles increases.
| Number of Triangles | Number of Rhombuses | Area of Triangles | Area of Rhombuses |
|---|---|---|---|
| 2 | 1 | 2
| base – height |
| 4 | 2 | 4
| 2
|
| 6 | 3 | 6
| 3
|
| 8 | 4 | 8
| 4
|
| 10 | 5 | 10
| 5
|
This table demonstrates a clear pattern. As the number of triangles increases, the number of rhombuses formed also increases proportionally. This pattern is evident in the area calculations, where the area of the rhombuses is always twice the area of the triangles used to create them. This relationship highlights the direct correlation between the number of triangles and the resulting rhombus.
Calculation Method: How Many Pattern Block Rhombuses Would 10 Triangles Create
To determine the number of rhombuses formed from a given number of pattern block triangles, we can utilize a straightforward calculation method. This method involves understanding the relationship between the number of triangles and the number of rhombuses that can be formed.
The Relationship Between Triangles and Rhombuses, How many pattern block rhombuses would 10 triangles create
Each rhombus is formed by combining two equilateral triangles. Therefore, to calculate the number of rhombuses, we simply need to divide the number of triangles by two.
Number of Rhombuses = Number of Triangles / 2
Calculation Example
Let’s apply this method to the example of 10 triangles.
- Identify the number of triangles: We have 10 triangles.
- Divide the number of triangles by 2: 10 triangles / 2 = 5 rhombuses.
Therefore, 10 triangles can form 5 rhombuses.
Application and Exploration
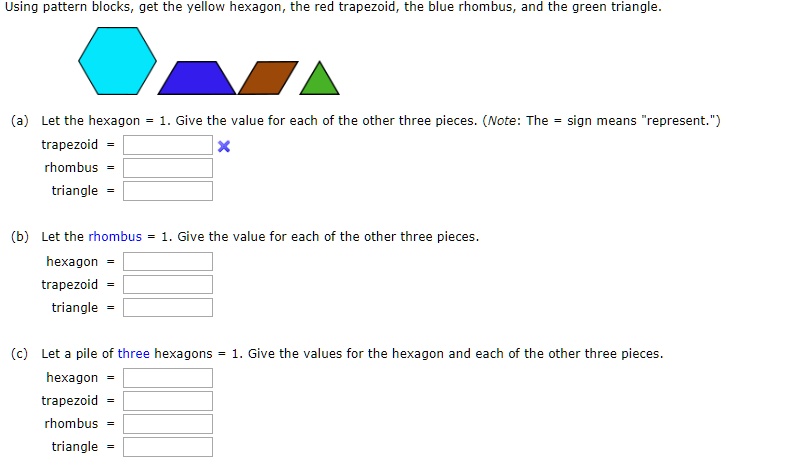
The conversion of triangles to rhombuses using pattern blocks opens up a world of possibilities for exploring geometric concepts and engaging in creative activities. This conversion forms the foundation for various pattern block explorations, offering a practical approach to understanding area equivalence and exploring the relationships between different shapes.
Area Equivalence
Understanding area equivalence is crucial in geometry. Area equivalence means that two shapes have the same area even though they may look different. The conversion of 10 triangles to 5 rhombuses demonstrates area equivalence. Each rhombus is equivalent in area to two triangles. This concept can be applied to various pattern block activities.
For instance, students can explore different combinations of shapes that have the same area, such as creating different designs with 5 rhombuses or 10 triangles, demonstrating that they have the same area. This hands-on approach helps students visualize and understand the concept of area equivalence in a concrete and engaging way.
Relationship Between Shapes
The relationship between the number of triangles and other shapes that can be formed using pattern blocks is fascinating. The conversion of triangles to rhombuses is a simple example of this relationship. This relationship can be further explored by considering other shapes like squares, trapezoids, and hexagons. For example, a square can be created using 4 triangles, a trapezoid using 3 triangles, and a hexagon using 6 triangles.
These relationships can be explored through activities where students are challenged to build different shapes using a specific number of triangles, helping them develop spatial reasoning and problem-solving skills.
Understanding the relationship between triangles and rhombuses in pattern blocks provides a valuable foundation for exploring more complex geometric concepts. This conversion process highlights the importance of area equivalence and demonstrates how shapes can be manipulated and transformed while preserving their underlying mathematical properties. As we delve deeper into the world of pattern blocks, we discover that seemingly simple shapes hold a wealth of mathematical knowledge, waiting to be unlocked by curious minds.
Quick FAQs
What is the relationship between a triangle and a rhombus in pattern blocks?
A rhombus is formed by two equilateral triangles placed side-by-side.
How does area apply to this conversion?
The area of a rhombus is equivalent to the combined area of the two triangles that form it.
What are some practical applications of this conversion?
This conversion can be used in pattern block activities to explore area, shape transformation, and problem-solving skills.
Can we form other shapes using pattern blocks?
Yes, pattern blocks can be used to create a wide variety of shapes, including squares, hexagons, and trapezoids.





